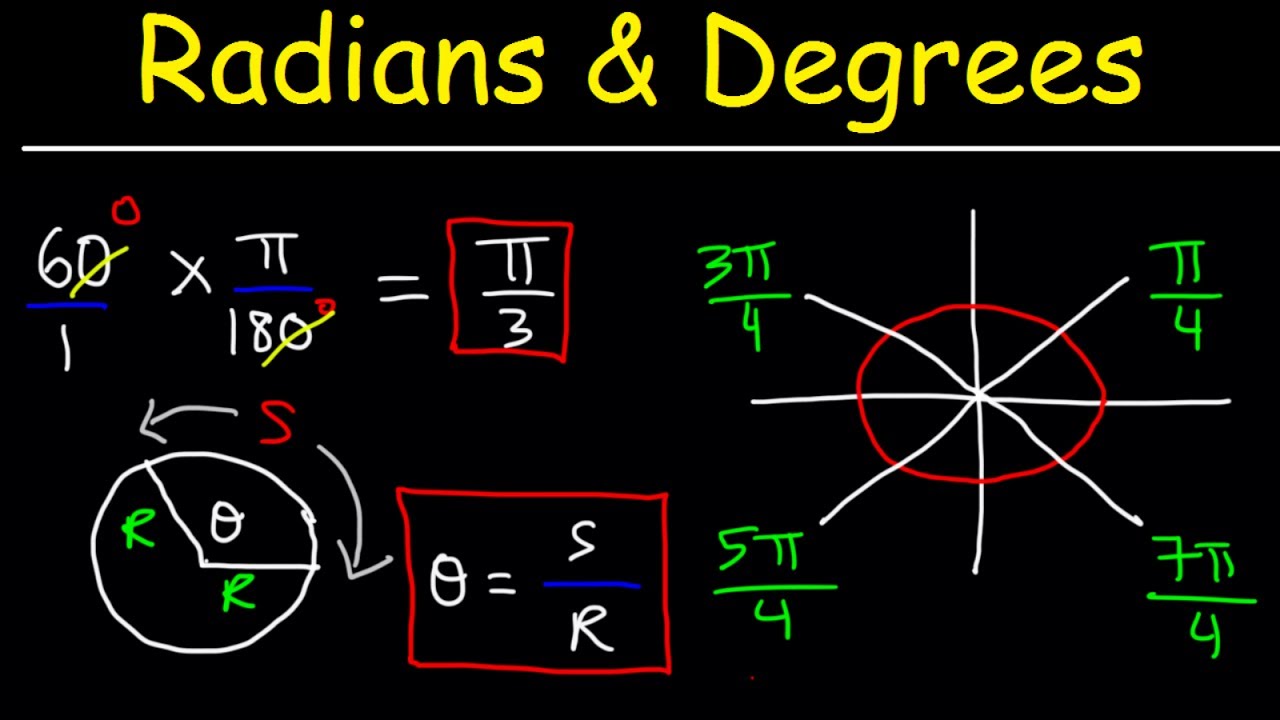
4 How does radian measure of an angle compare to the degree measure?2 π radians = 360 ∘ π radians = 360 ∘ 2 = 180 ∘ 1 radian = 180 ∘ π ≈ 573 ∘ 2 π radians = 360 ∘ π radians = 360 ∘ 2 = 180 ∘ 1 radian = 180 ∘ π ≈ 573 ∘ Note that when an angle is described without a specific unit, it refers to radian measureReference Angle and Quadrant Calculator This online calculator finds the reference angle and the quadrant of a trigonometric a angle in standard positionThe reference angle is defined as the acute angle between the terminal side of the given angle and the x axis The given angle may be in degrees or radians
Radians And Degrees Youtube
π 2 radian angle in standard position
π 2 radian angle in standard position-We have a special type of angle in standard position whose terminal side lies on an axis, a quadrantal angle This type of angle can have a measure of 0°, 90°, 180°, 270° or 360° See Figure \(\PageIndex{7}\) Figure \(\PageIndex{7}\) Quadrantal angles are angles in standard position whose terminal side lies along an axisRadian to degree conversion Multiply radians by 180 ° π radian First convert the angle from 15 π 4 radians into degrees using above formula 15 π 4 = 15 π 4 × 180 ° π = 675 ° Now, 675 ° = 360 ° 215 ° The sketch of the angle 15 π 4 in standard position is given below



T1 1d To Find Coterminal Angles Ppt Download
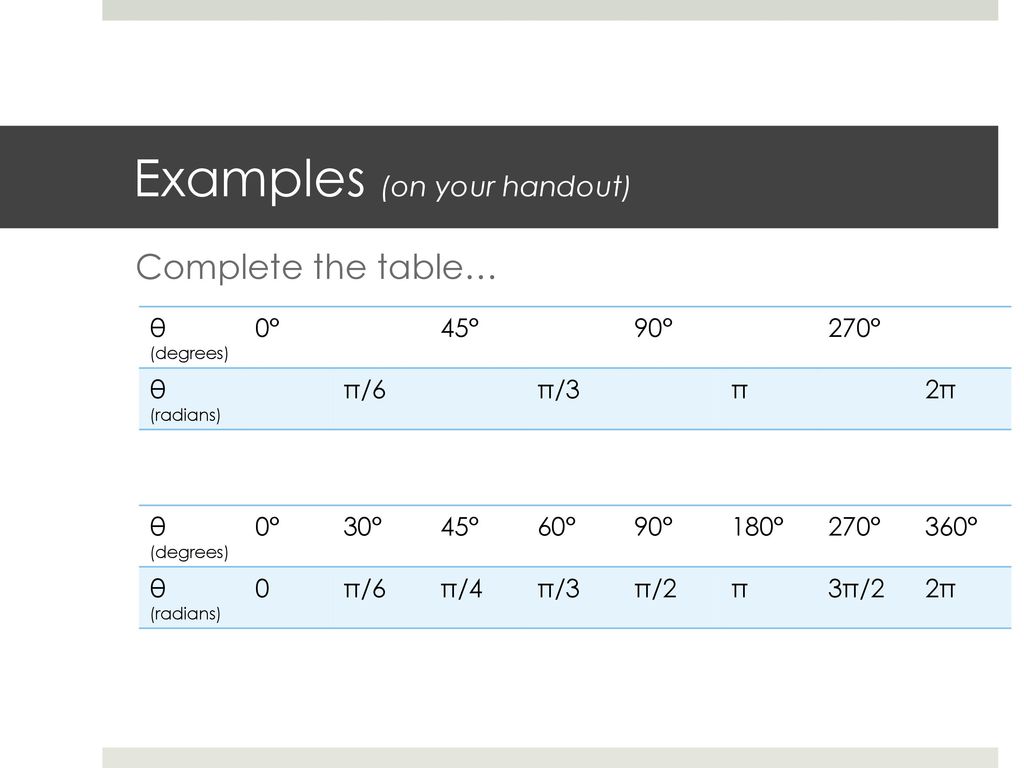
Example 14 Draw the following angles in standard position 5π 2 −135 3 (radians) When changing from degrees to radians or vice versa just remember that degrees/360 is the same fraction of a circle as radians/2π So if an angle is x degrees and y radians, then x 360 = y 2π Solving we have the formulas x = 180 π y and y = πx 180Draw the following angles in standard position 5π 2 −135 3 (radians) When changing from degrees to radians or vice versa just remember that degrees/360 is the same fraction of a circle as radians/2π So if an angle is x degrees and y radians, then x 360 = y 2π Solving we have the formulas x = 180 π y and y = πx 1801 Sketch an angle in standard position of 4 radians 2 Convert the angle measure to radian measure a 78 b 54° 3 The circular blade on a saw has a diameter of 725 inches and rotates at 4800 revolutions per minute Find the angular speed of the blade both in radians per second and as a multiple of π 4
Jan 21, 21 · Draw an angle in standard position with the given measure If the angle measure is not between 0 and 2 π or between 0 ∘ and \ (360^ {\circ\), state the coterminal angle that is within that interval 6) 30 ∘ 7) 300 ∘ 8) − 80 ∘ 9) 135 ∘2 Convert from radians to degrees a) 5 4 π b) 17 6 π c) 7 6 π − d)2radians Coterminal Angles Two angles, when in standard position, have the same terminal angle 3 Find two coterminal angles for the given angle A) 115 ° B) 4 π C) 3 2 π − D) 440 ° Complementary and Supplementary Angles Complementary Angles – The sum of theirThe angles that bisect the other quadrants are π/2 π/4 = 3π/4 radians, π π/4 = 5π/4 radians and 3π/2 π/4 = 7π/4 radians The angle one third of the way through the first quadrant is one twelfth of a revolution, that is, (π/2)/3 = 2π/12 = π/6 radians Double π/6 is twothirds of the way through the first quadrant, or one sixth
Angles in standard position via radians as well Sketch the following angles on the board (2π, π, π/2, 3π/2 radians) before examining the next example Example Draw and label each angle in standard position (SHOW figure 415 and table 41 on pg 467) θ = α = α = β = θ = Two angles with the same initial and terminal sides (butAug 04, 17 · The terminal side is in the third quadrant pi ~~ 314 so the terminal side of4 is past the negative xaxis (3pi)/2 = 3/2 * pi ~~ 3/2 * 3 = 45 so the terminal side of 4 is not in the fourth quadrant If you want to be a little more precise, note that the pi/4 angle in Quadrant 3 is (5pi)/4 ~~ 15/4 = 375, so the terminal side of 4 is between those of (5pi)/4 and (3pi)/2Recall that the symbol represents the real number constant which is the ratio of the circumference of a circle to its diameter Its value is approximately Since the circumference of the unit circle is 2 it follows that the radian measure of an angle of one revolution is 2The radian measure of an angle whose terminal side is along the negative xaxis is



Angles Precalculus Ii


Radian And Degree Measure Ppt Download
This online calculator finds the quadrant of an angle in standard position The given angle may be in degrees or radians Use of calculator to Find the Quadrant of an Angle 1 Enter the angle in Degrees top input example 1250 in Radians second input as a fraction of π Example 27/5 π or 12 πStandard position is when Measure angles in standard position using degree measure and radian measure DRAFT 11th 12th grade 43 times which picture shows an angle of π/6 radians?470 Chapter 9 Trigonometric Ratios and Functions 92 Lesson WWhat You Will Learnhat You Will Learn Draw angles in standard position Find coterminal angles Use radian measure Drawing Angles in Standard Position In this lesson, you will expand your study of angles to include angles


Question Video Using The Pythagorean Identities And Unit Circle To Solve An Equation In One Variable Nagwa



Radian And Degree Measure Ppt Download
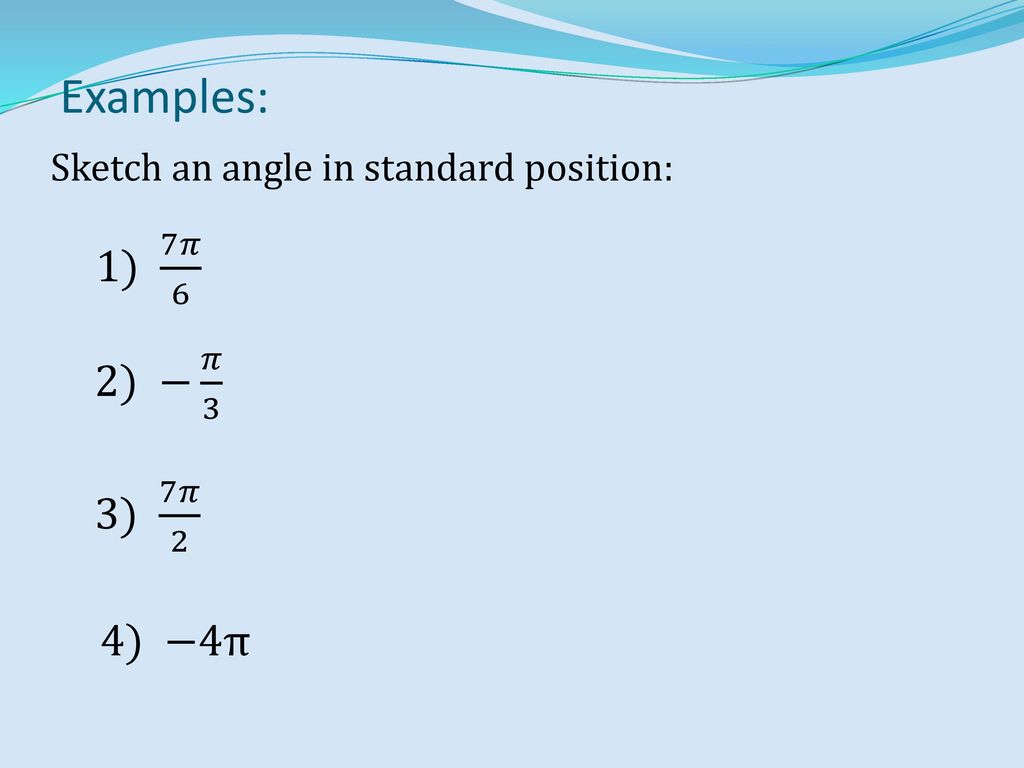
PRACTICE Drawing Angles in Radians and Exact Values For each of the following a Draw the angle given in standard position b Find the related acute angle c Draw and label the special triangle d Use the triangle to state the trig ratios (primary on the left column, secondary on the right column) 1 3 4 π 2 4 3 π 3 6 π 4 5 3 π 5 5 4 πAn angle is in standard position when its vertex is at the origin 2 π °= radians All other special angles shown are multiples of theses angles Arc Length and Area of a Sector s and area A of a sector with radius r and central angle θ (measured in radians) are as followsQuadrantal Angle An angle in standard position whose terminal side lies along one of the axes Radian A unit of measure for angles 2Π radians equals one revolution and 360 degrees Ray A part of a line with a fixed endpoint on one end that extends without bound in


Pplato Flap Math 3 2 Polar Representation Of Complex Numbers


Radian And Degree Measure Ppt Download
One half rotation is 180° or π radians One quarter rotation is 90° or π_ 2 radians One eighth rotation is 45° or π_ 4 radians Many mathematicians omit units for radian measures For example, 2_π 3 radians may be written as _2π 3 Angle measures without units are considered to be in radians Link the Ideas radian • one radian is theTrigonometric Ratios and Angle Measures Topics 1 Angle in standard position 2 Coterminal angles 3 Reference angle 4 Find the exact value of trigonometric ratios 5 ASTC rule in trigonometry (All Students Take Calculus) 6 Unit circle 7 Converting between degrees and radians 8 Trigonometric ratios of angles in radians 9 RadianCoterminal angles are angles in standard position (angles with the initial side on the positive x axis) that have a common terminal side For example 30 ° , − 330 ° and 390 ° are all coterminal To find a positive and a negative angle coterminal with a given angle, you can add and subtract 360 ° if the angle is measured in degrees or 2 π if the angle is measured in radians
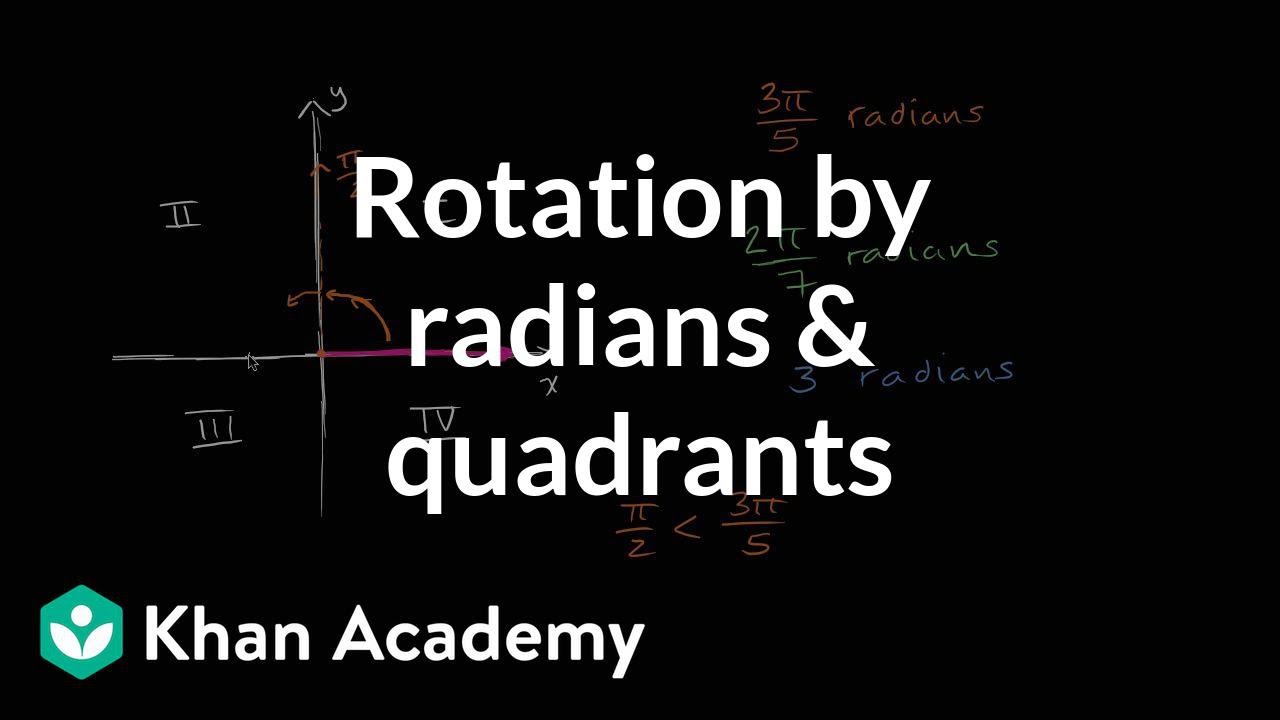


Radian Angles Quadrants Video Radians Khan Academy



Day 9 Hw 1 And 2 Find A Coterminal Angle Between 0 And 2 Pi Youtube
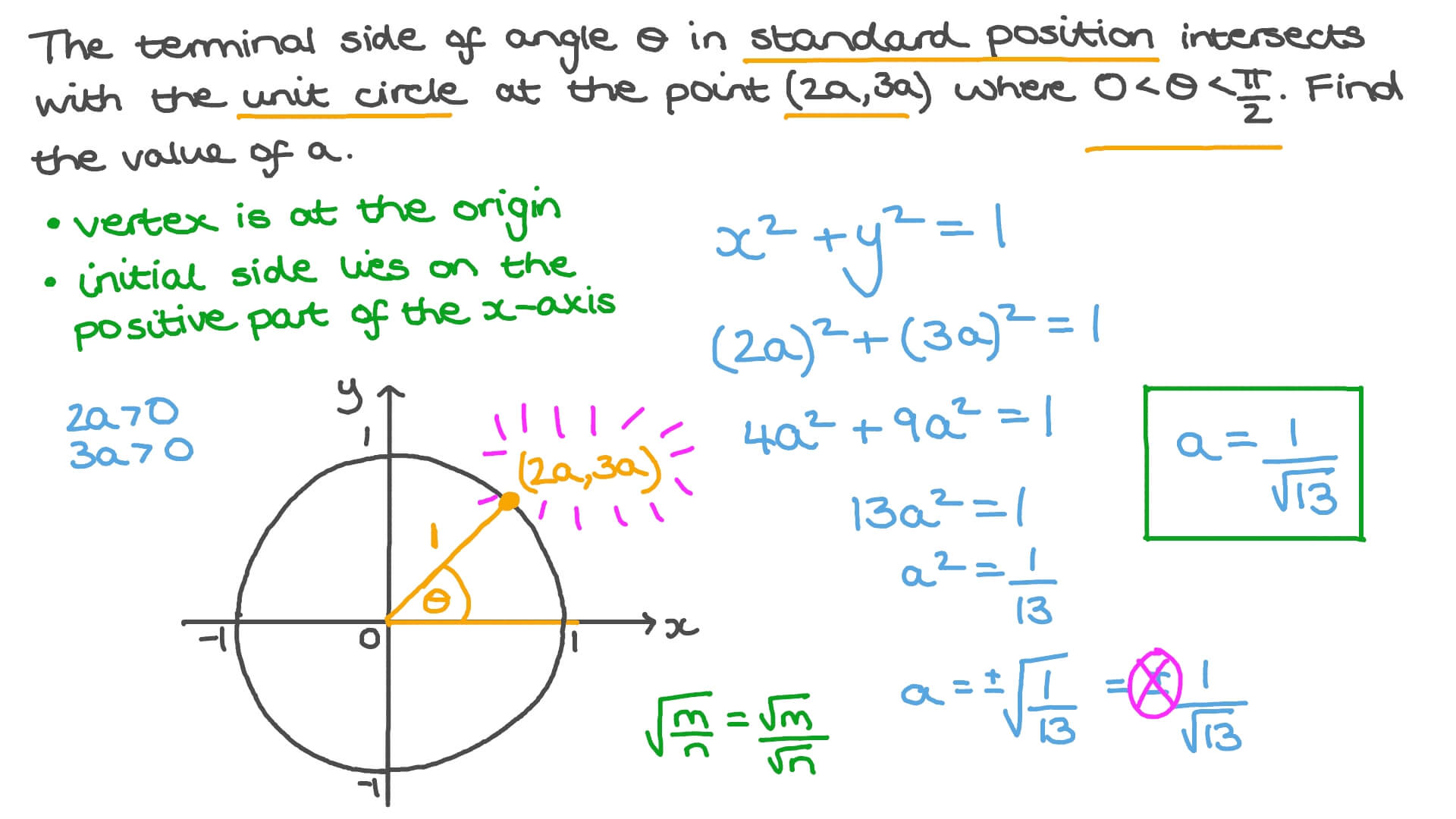
(Through what quadrant does the terminal side pass when the angle is in standard position?) Solution Observe 3π 6 = π 2 < 5π 6 < 6π 6 =π , so 5π 6 is in Quadrant IIAngles Definition A quadrantal angle is an angle in standard position whose terminal ray lies along one of the axes Examples of quadrantal angles include, 0, π/2, π, and 3π/ 2Oct 13, 11 · Draw an angle with the given measure in standard position 1) 110o 2) 450o 3) 3π/2 (Hint change to degrees first) Find one positive angle and one negative angle that are coterminal with the given angles 4) 87o 5) 1o Apr 71250 PM 6) Let (3,4) be a point on the terminal side of an angle θ in standard position
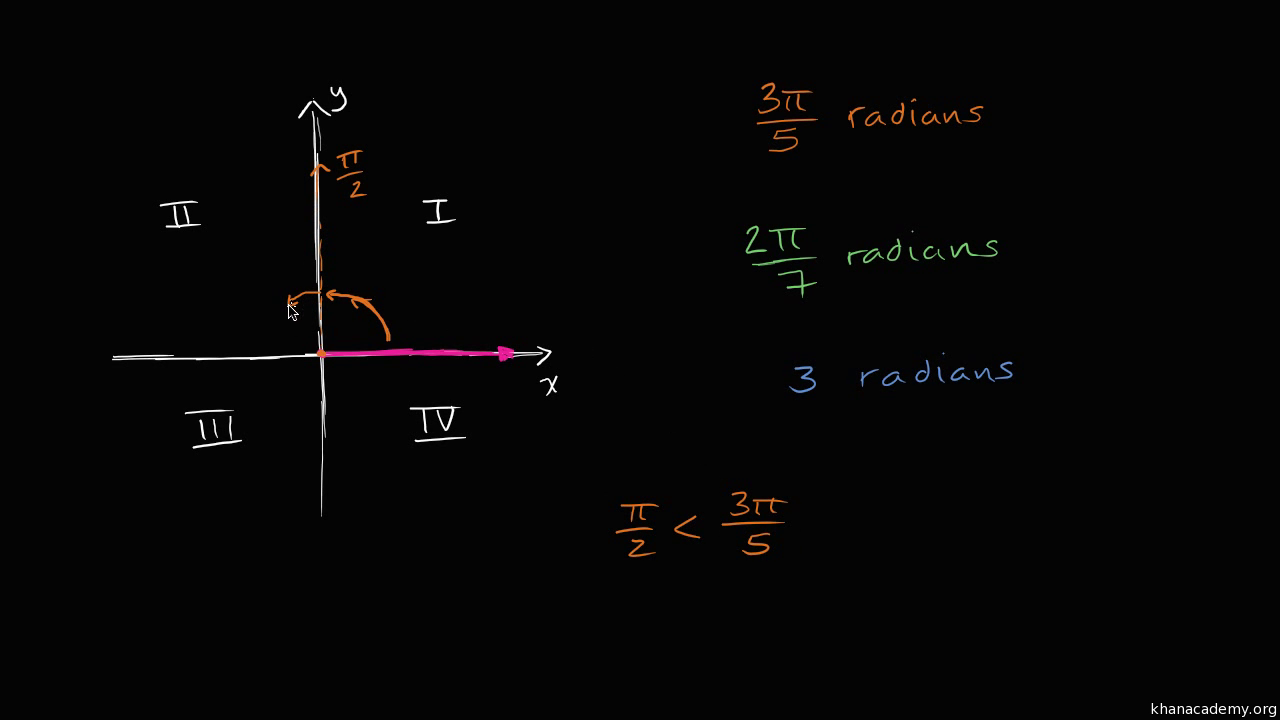


Radian Angles Quadrants Video Radians Khan Academy
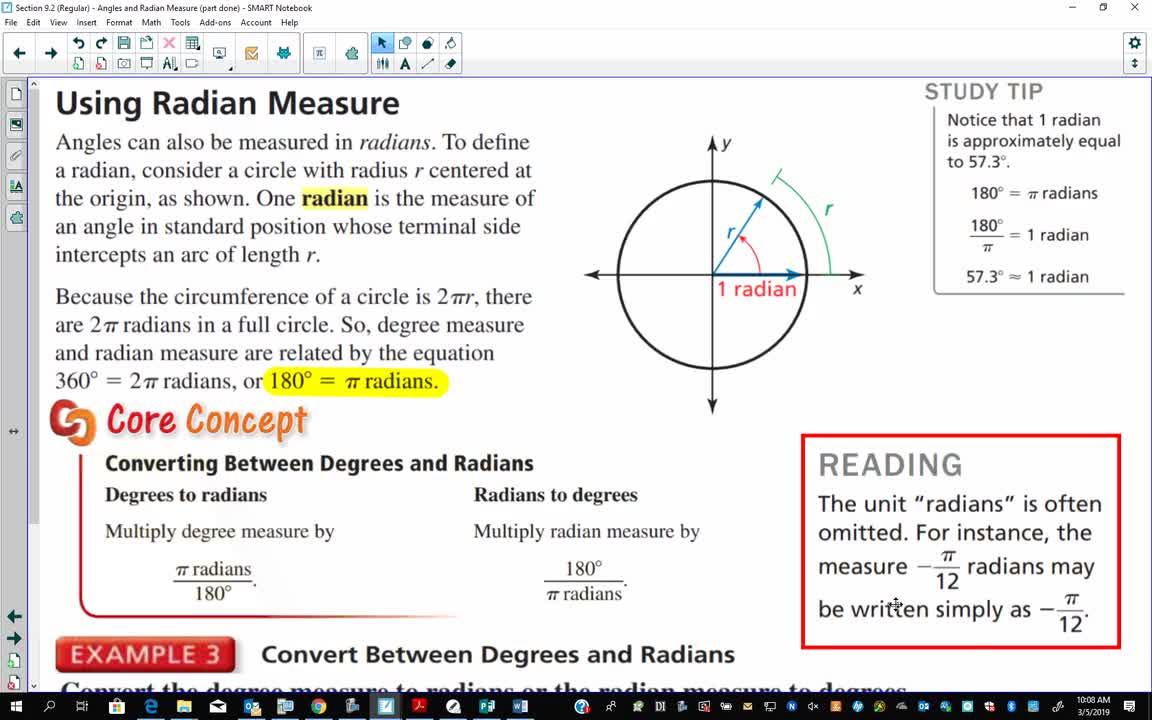


Regular Algebra 2 Section 9 2 Part 2 21 Minutes Yankton High School


